Providence Salumu A Haskell Compiler

A Haskell Compiler

A Haskell Compiler

Structure of Lecture

Will first give an overview of the intuitive ideas behind the compiler.

Then will go through how GHC works in terms of traditional compiler stages:

Why is Haskell difficult

Haskell is seen as a difficult language to understand from a compilation perspective.

There are some good reasons for this:

But one reason (an annoying one) is the use of Jargon

Terminology 101

Two good resources for dealing with unknown terminology:

Lets cover some of the terminology now:

Yes, closures, thunks and continuations are all very similar. One implementation can capture them all, however the terminology is used to capture the different use cases.

Statistics of GHC

Pipeline of GHC

Core

We will start though with a quick look at Core, the main intermediate language used by GHC:

variables, literals, let, case, lambda abstraction, application

Useful tool for viewing Core:

cabal install ghc-core

Core in one slide

data Expr b -- "b" for the type of binders, 
  = Var    Id
  | Lit   Literal
  | App   (Expr b) (Arg b)
  | Lam   b (Expr b)
  | Let   (Bind b) (Expr b)
  | Case  (Expr b) b Type [Alt b]

  | Type  Type
  | Cast  (Expr b) Coercion
  | Coercion Coercion

  | Tick  (Tickish Id) (Expr b)

data Bind b = NonRec b (Expr b)
            | Rec [(b, (Expr b))]

type Arg b = Expr b

type Alt b = (AltCon, [b], Expr b)

data AltCon = DataAlt DataCon | LitAlt  Literal | DEFAULT

Graph Reduction

The way that lazy functional languages like Haskell are implemented is through a technique called graph reduction

Its best to use the graph reduction model as an intuitive way to think about how Haskell is evaluated, the actual way GHC implements Haskell is pretty close to how an imperative language works.

f g = let x = 2 + 2
      in (g x, x)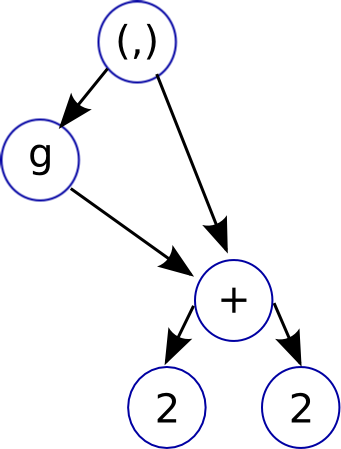

Terminology 102

Terminology 103

Front End: Haskell -> Core

Lets now look at how Haskell is compiled to Core.

Terminology 104

Functions -> Core

Haskell

idChar :: Char -> Char
idChar c = c

id :: a -> a
id x = x

idChar2 :: Char -> Char
idChar2 = id

Core

idChar :: GHC.Types.Char -> GHC.Types.Char
[GblId, Arity=1, Caf=NoCafRefs]
idChar = \ (c :: GHC.Types.Char) -> c

id :: forall a. a -> a
id = \ (@ a) (x :: a) -> x

idChar2 :: GHC.Types.Char -> GHC.Types.Char
idChar2 = id @ GHC.Types.Char

Functions -> Core

Haskell

map :: (a -> b) -> [a] -> [b]
map _ []     = []
map f (x:xs) = f x : map f xs

Core

map :: forall a b. (a -> b) -> [a] -> [b]
map =
  \ (@ a) (@ b) (f :: a -> b) (xs :: [a]) ->
    case xs of _ {
      []     -> GHC.Types.[] @ b;
      : y ys -> GHC.Types.: @ b (f y) (map @ a @ b f ys)
    }

Data -> Core

Haskell

data Maybe a = Nothing | Just a

none = Nothing
some = Just (1 :: Int)

Core

none :: forall a. Maybe a
none = Nothing

n :: GHC.Types.Int
n = GHC.Types.I# 1

some :: Maybe GHC.Types.Int
some = Just @ GHC.Types.Int n

Handling where

Haskell

dox :: Int -> Int
dox n = x * x
    where x = (n + 2) * 4

Core

dox :: GHC.Types.Int -> GHC.Types.Int
dox =
  \ (n :: GHC.Types.Int) ->
    let {
      x :: GHC.Types.Int
      x =
        GHC.Num.* @ GHC.Types.Int GHC.Num.$fNumInt
          (GHC.Num.+ @ GHC.Types.Int GHC.Num.$fNumInt n (GHC.Types.I# 2))
          (GHC.Types.I# 4) }

    in GHC.Num.* @ GHC.Types.Int GHC.Num.$fNumInt x x

Patterns & Guards

Haskell

iff :: Bool -> a -> a -> a
iff True  x _ = x
iff False _ y = y

Core

iff :: forall a. GHC.Bool.Bool -> a -> a -> a
iff =
  \ (@ a) (d :: GHC.Bool.Bool) (x :: a) (y :: a) ->
    case d of _
      GHC.Bool.False -> y
      GHC.Bool.True  -> x

Sharing & Updating

Haskell

sum100 :: Int -> Int
sum100 n = foldr (+) 0 [1..100]

Core

-- Unoptimized
sum100n = \ (n :: Int) -> * n (foldr (I# 0) (enumFromTo (I# 1) (I# 100)))

-- Optimized
sum100n = \ (n :: Int) -> GHC.Base.timesInt n sum100n1

sum100n1 = case $wgo 1 of r { __DEFAULT -> GHC.Types.I# r }

$wgo :: Int# -> Int#
$wgo = \ (w :: Int#) ->
    case w of w'
      __DEFAULT -> case $wgo (GHC.Prim.+# w' 1) of r
                      __DEFAULT -> GHC.Prim.+# w' r
      100 -> 100

Partial Evaluation -> Core

Haskell

add :: Int -> Int -> Int
add x y = x + y

add2 :: Int -> Int
add2 = add 2

Core (unoptimized)

add :: GHC.Types.Int -> GHC.Types.Int -> GHC.Types.Int
add =
  \ (x :: GHC.Types.Int) (y :: GHC.Types.Int) ->
    GHC.Num.+ @ GHC.Types.Int GHC.Num.$fNumInt x y

x :: GHC.Types.Int
x = GHC.Types.I# 2

add2 :: GHC.Types.Int -> GHC.Types.Int
add2 =
  \ (y :: GHC.Types.Int) ->
    GHC.Num.+ @ GHC.Types.Int GHC.Num.$fNumInt x y

Partial Evaluation -> Core

Haskell

add :: Int -> Int -> Int
add x y = x + y

add2 :: Int -> Int
add2 = add 2

Core (optimized)

add :: GHC.Types.Int -> GHC.Types.Int -> GHC.Types.Int
Hs2Core.add = GHC.Base.plusInt

x :: GHC.Types.Int
x = GHC.Types.I# 2

add2 :: GHC.Types.Int -> GHC.Types.Int
add2 = GHC.Base.plusInt x

(+) -> Core

The function GHC.Base.plusInt is implemented as:

+ :: Int -> Int -> Int
+ = \ a b -> case a of _
                 I# a_ -> case b of _
                              I# b_ -> I# (GHC.Prim.+# a_ b_)

Type Classes -> Core

Haskell

typeclass MyEnum a where
   toId  :: a -> Int
   fromId :: Int -> a

instance MyEnum Int where
   toId = id
   fromId = id

instance (MyEnum a) => MyEnum (Maybe a) where
   toId (Nothing) = 0
   toId (Just n)  = 1 + toId n
   fromId 0       = Nothing
   fromId n       = Just (fromId $ n - 1)

Core

toId :: forall a. MyEnum a => a -> GHC.Types.Int
toId =
  \ (@ a) (d :: MyEnum a) ->
    case d of _ { D:MyEnum f1 _ -> f1 }

fromId :: forall a. MyEnum a => GHC.Types.Int -> a
fromId =
  \ (@ a) (d :: MyEnum a) ->
    case d of _ { D:MyEnum _ f2 -> f2 }

Type Classes -> Core

Core

$fMyEnumInt :: MyEnum GHC.Types.Int
$fMyEnumInt = D:MyEnum @ GHC.Types.Int (id @ GHC.Types.Int) (id @ GHC.Types.Int)

$fMyEnumMaybe :: forall a. MyEnum a => MyEnum (Maybe a)
$fMyEnumMaybe =
  \ (@ a) ($dMyEnum_arR :: MyEnum a) ->
    D:MyEnum @ (Maybe a_acF)
      ($fMyEnumMaybe_$ctoId @ a $dMyEnum_arR)
      ($fMyEnumMaybe_$cfromId @ a $dMyEnum_arR)

$fMyEnumMaybe_$ctoId :: forall a. Hs2Core.MyEnum a => Hs2Core.Maybe a -> GHC.Types.Int
$fMyEnumMaybe_$ctoId =
  \ (@ a) ($dMyEnum_arR :: MyEnum a) (ds :: Maybe a) ->
    case ds of _
      Nothing -> GHC.Types.I# 0
      Just n  -> case toId @ a $dMyEnum_arR n of _ 
                    GHC.Types.I# y -> GHC.Types.I# (GHC.Prim.+# 1 y)

IO -> Core

newtype IO a = IO (State# RealWorld -> (# State# RealWorld, a #))

Haskell

f :: IO ()
f = do
   putStrLn "Hello World"
   putStrLn "What's up today?"

IO -> Core

Core (Unoptimized)

g :: GHC.Types.IO ()
g =
  GHC.Base.>> @ GHC.Types.IO GHC.Base.$fMonadIO @ () @ ()
    (System.IO.putStrLn (GHC.Base.unpackCString# "Hello World"))
    (System.IO.putStrLn (GHC.Base.unpackCString# "What's up today?"))

Core (optimized)

f :: GHC.Prim.State# GHC.Prim.RealWorld -> (# GHC.Prim.State# GHC.Prim.RealWorld, () #)
f =
  \ (world :: GHC.Prim.State# GHC.Prim.RealWorld) ->
    case hPutStr2 stdout f1 True world of _
       (# new_world, _ #) -> hPutStr2 stdout f2 True new_world

f1 :: [GHC.Types.Char]
f2 = GHC.Base.unpackCString# "Hello World"

f2 :: [GHC.Types.Char]
f1 = GHC.Base.unpackCString# "What's up today?"

Lazy Evaluation -> Core

Haskell

foldl :: (a -> b -> a) -> a -> [b] -> a

foldl' :: (a -> b -> a) -> a -> [b] -> a

forcee :: a -> b -> b
forccee = seq

Core

foldl = \ (f :: a -> b -> a) (z :: a) (d :: [b]) ->
    case d of _
      [] -> z;
      : x xs -> foldl f (f z x) xs

foldl' = \ (f :: a -> b -> a) (z :: a) (d :: [b]) ->
    case d of _
      [] -> z;
      : x xs ->
        case f z x of z'
           __DEFAULT -> foldl' b f z' xs

forccee = \ (x :: a) (y :: b) -> case x of _ { __DEFAULT -> y }

Core Summary

Middle of GHC: Core -> Core

Hopefully you have a decent idea of how Haskell is reduced to Core now. Once we have the Core IR we can do a lot of optimization work:

Some standard optimisations

Rest of the optimisations GHC does are fairly specific to a functional language. Lets look at a few of them.

Fun Fact: Estimated that functional languages gain 20 - 40%
improvement from inlining Vs. imperative languages which gain 10 - 15%

STG Code

Strictness & Unboxing

Naive compilation of factorial

Consider this factorial implementation in Haskell:

fac :: Int -> Int -> Int
fac a 0 = a
fac a n = fac (n*a) (n-1)

STG

fac = \ a n -> case n of 
                   I# n# -> case n# of
                                0# -> a
                                _  -> let one = I# 1;
                                          x = n - one
                                          y = n * a;
                                      in  fac y x

GHC with strictness analysis and unboxing

If we compile in GHC with optimisations turned on:

one = I# 0#

-- worker :: Int# -> Int# -> Int#
$wfac = \ a# n# -> case n# of
                     0#  -> a#
                     n'# -> case (n'# -# 1#) of
                                m# -> case (n'# *# a#) of
                                           x# -> $wfac x# m#

-- wrapper :: Int -> Int -> Int
fac = \ a n -> case a of
                    I# a# -> case n of
                                 I# n# -> case ($wfac a# n#) of
                                              r# -> I# r#

SpecConstr

The idea of the SpecConstr pass is to extend the strictness and unboxing from before but to functions where arguments aren't strict in every code path.

Consider this Haskell function:

drop :: Int -> [a] -> [a]
drop n []     = []
drop 0 xs     = xs
drop n (x:xs) = drop (n-1) xs

So we get this code in STG:

drop n xs = case xs of
              []     -> []
              (y:ys) -> case n of 
                          I# n# -> case n# of
                                      0 -> xs
                                      _ -> drop (I# (n# -# 1#)) ys

SpecConstr

The SpecConstr pass takes advantage of this to create a specialised version of drop that is only called after we have passed the first check where we may not want to evaluate n.

Basically we aren't specialising the whole function but a particular branch of it that is heavily used (ie. recursive)

drop n xs = case xs of
              []     -> []
              (y:ys) -> case n of 
                          I# n# -> case n# of
                                      0 -> xs
                                      _ -> drop' (n# -# 1#) ys

-- works with unboxed n
drop' n# xs = case xs of
               []     -> []
               (y:ys) -> case n# of
                           0# -> xs
                           _  -> drop (n# -# 1#) ys

Backend: Core -> Assembly

Final stage of GHC is compiling Core to an executable. The backend is in two parts:

Cmm is a low level imperative language used in GHC. Basically a very simple C like language. Just enough to abstract away hardware registers, call conventions:

STG -> Cmm

So what has been handled and what is left to handle?

The STG Machine

The way the operational semantics of the STG language is defined is by an abstract machine called 'The STG Machine'.

STG Machine -> Cmm

Lets just look at some of the details of the code generator. The final backends are all pretty straight forward (think simple C compiler). The important parts of the code generator are:

Closure Representation

The STG machine represents function and data values as heap allocated closures. Delayed computations, thunks, are also represented by closure objects.

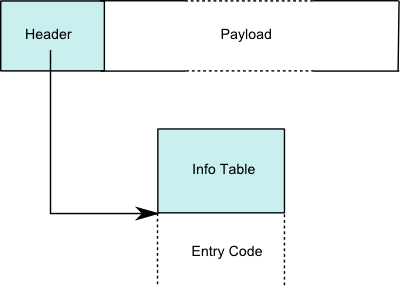
In GHC all Heap objects have the same layout:

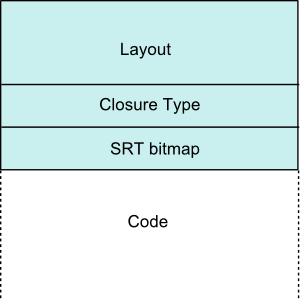
Closure Info Table

Closure Representation

Closure Representation

Heap & Stack Layout

GHC has a very nice uniform way of managing the heap and stack.

Terminology 105

Call Convention

id' x = x
A_idzq_entry()
    R1 = R2;
    jump stg_ap_0_fast ();
stg_ap_0_fast { 
  ENTER();
}

#define ENTER()
  // ...
  case
    FUN,
    // ...
    PAP:     { jump %ENTRY_CODE(Sp(0)); }
    default: { info = %INFO_PTR(UNTAG(R1)); jump %ENTRY_CODE(info); }

Call Convention

Calling a known Haskell function:

Haskell

x :: Int -> Int
x z = (+) 2 (id z)

Cmm

I64[Hp - 8] = spH_info;                  // create thunk on heap
I64[Hp + 0] = R2;                        // R2 = z, store argument in closure
R2 = stg_INTLIKE_closure+289;            // first argument (static closure for '2')
R3 = Hp - 16;                            // second argument (closure pointer)
jump base_GHCziBase_plusInt ();          // call (+) function

Call Convention

What happens though when we are calling an unknown function?

Haskell

unknown_app :: (Int -> Int) -> Int -> Int
unknown_app f x = f x

Cmm

unknownzuapp_entry() {
    cnO:
        R1 = R2;
        Sp = Sp + 4;
        jump stg_ap_p_fast ();
}

Data Constructors

Haskell

10

Cmm

section "data" {
    A_ten_closure:
        const ghczmprim_GHCziTypes_Izh_static_info;
        const 10;
}

Pointer Tagging

Data Constructors

Haskell

build_just :: a -> Maybe a
build_just x = Just x

Cmm

buildzujust_entry()
    crp:
        Hp = Hp + 16;
        if (Hp > HpLim) goto crt;                        // Allocte heap space
        I64[Hp - 8] = base_DataziMaybe_Just_con_info;    // Just constructor tag
        I64[Hp + 0] = R2;                                // store x in Just
        R1 = Hp - 6;                                     // setup R1 as argument to continuation
                                                         //     (we do '- 6' and not '8' to set the pointer tag)
        jump (I64[Sp + 0]) ();                           // jump to continuation
    cru:
        R1 = buildzujust_closure;
        jump stg_gc_fun ();
    crt:
        HpAlloc = 16;
        goto cru;
}

Case Statements

Haskell

mycase :: Maybe Int -> Int
mycase x = case x of Just z -> z; Nothing -> 10

Cmm

mycase_entry()                          // corresponds to forcing 'x'
    crG:
        R1 = R2;                        // R1 = 'x'
        I64[Sp - 8] = src_info;         // setup case continuation
        Sp = Sp - 8;
        if (R1 & 7 != 0) goto crL;      // check pointer tag to see if x eval'd
        jump I64[R1] ();                // x not eval'd, so eval
    crL:
        jump src_info ();               // jump to case continuation
}

src_ret()                               // case continuation
    crC:
        v::I64 = R1 & 7;                // get tag bits of 'x' and put in local variable 'v'
        if (_crD::I64 >= 2) goto crE;   // can use tag bits to check which constructor we have
        R1 = stg_INTLIKE_closure+417;   // 'Nothing' case
        Sp = Sp + 8;                    // pop stack
        jump (I64[Sp + 0]) ();          // jump to continuation ~= return
    crE:
        R1 = I64[R1 + 6];               // get 'z' thunk inside Just
        Sp = Sp + 8;                    // pop stack
        R1 = R1 & (-8);                 // clear tags on 'z'
        jump I64[R1] ();                // force 'z' thunk
}

Graph Reduction: Thunks, Updates & Indirections

Lets take a look at the code for the (x + 1) thunk:

build_data :: Int -> Maybe Int
build_data x = Just (x + 1)

Cmm

sus_entry()
    cxa:
        if (Sp - 24 < SpLim) goto cxc;
        I64[Sp - 16] = stg_upd_frame_info;  // setup update frame (closure type)
        I64[Sp -  8] = R1;                  // set thunk to be updated (payload)
        I64[Sp - 24] = sut_info;            // setup continuation (+) continuation
        Sp = Sp - 24;                       // increase stack
        R1 = I64[R1 + 16];                  // grab 'x' from environment
        if (R1 & 7 != 0) goto cxd;          // check if 'x' is eval'd
        jump I64[R1] ();                    // not eval'd so eval
    cxc: jump stg_gc_enter_1 ();
    cxd: jump sut_info ();                  // 'x' eval'd so jump to (+) continuation
}

sut_ret()
    cx0:
        Hp = Hp + 16;
        if (Hp > HpLim) goto cx5;
        v::I64 = I64[R1 + 7] + 1;           // perform ('x' + 1)
        I64[Hp - 8] = ghczmprim_GHCziTypes_Izh_con_info; // setup Int closure
        I64[Hp + 0] = v::I64;               // setup Int closure
        R1 = Hp - 7;                        // point R1 to computed thunk value (with tag)
        Sp = Sp + 8;                        // pop stack
        jump (I64[Sp + 0]) ();              // jump to continuation ('stg_upd_frame_info')
    cx6: jump stg_gc_enter_1 ();
    cx5:
        HpAlloc = 16;
        goto cx6;
}

Graph Reduction: Thunks & Updates

I64[Sp - 16] = stg_upd_frame_info;  // setup update frame (closure type)
I64[Sp -  8] = R1;                  // set thunk to be updated (payload)

RTS & Garbage Collection

RTS & Garbage Collection

RTS & Garbage Collection

Bringing it all home

No lecture on Compilers is complete without assembly code!

add :: Int -> Int -> Int
add x y = x + y + 2
A_add_info:
.LcvZ:
    leaq -16(%rbp),%rax
    cmpq %r15,%rax
    jb .Lcw1
    movq %rsi,-8(%rbp)
    movq %r14,%rbx
    movq $sul_info,-16(%rbp)
    addq $-16,%rbp
    testq $7,%rbx
    jne sul_info
    jmp *(%rbx)
.Lcw1:
    movl $A_add_closure,%ebx
    jmp *-8(%r13)

sul_info:
.LcvS:
    movq 8(%rbp),%rax
    movq 7(%rbx),%rcx
    movq %rcx,8(%rbp)
    movq %rax,%rbx
    movq $suk_info,0(%rbp)
    testq $7,%rbx
    jne suk_info
    jmp *(%rbx)

suk_info:
.LcvK:
    addq $16,%r12
    cmpq 144(%r13),%r12
    ja .LcvP
    movq 7(%rbx),%rax
    addq $2,%rax
    movq 8(%rbp),%rcx
    addq %rax,%rcx
    movq $ghczmprim_GHCziTypes_Izh_con_info,-8(%r12)
    movq %rcx,0(%r12)
    leaq -7(%r12),%rbx
    addq $16,%rbp
    jmp *0(%rbp)
.LcvP:
    movq $16,184(%r13)
.LcvQ:
    jmp *-16(%r13)

Finished!

Resources & References

Here are some resources to learn about GHC, they were also used to create these slides:

General:

Execution Model:

Resources & References

Here are some resources to learn about GHC, they were also used to create these slides:

RTS:

Haskell Features:

Resources & References

Here are some resources to learn about GHC, they were also used to create these slides:

GHC Internals:

Optimization:

Providence Salumu